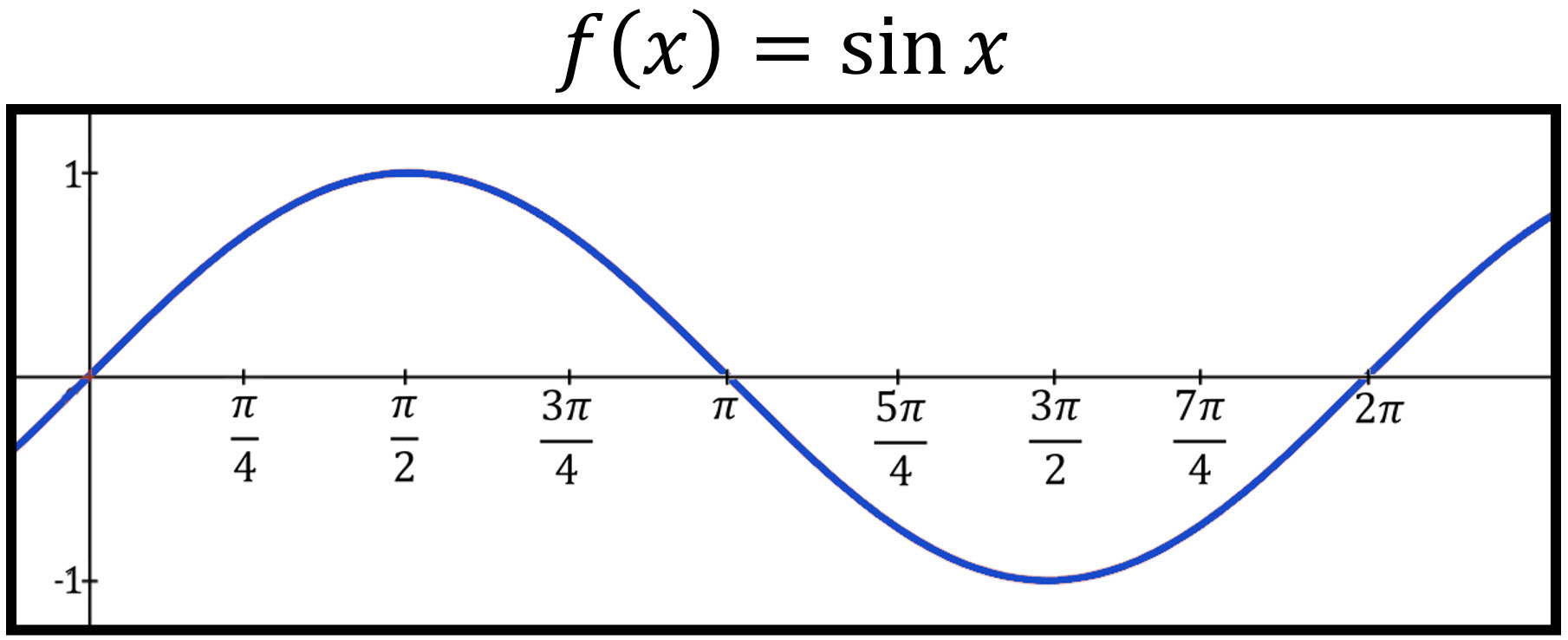
Calculus Using the first and second derivative, sketch the graph of f(x) = sin(x) cos(x)Here is the graph of y = sin x The height of the curve at every point is the line value of the sine In the language of functions, y = sin x is an odd function It is symmetrical with respect to the origin sin (−x) = −sin x y = cos x is an even function The independent variable x is the radian measure x may be any real number We may imagine the unit circle rolled out, in both · f (x) = cosx ⇒ f '(x) = −sinx This is the graph of y = f (x) And this is a graph of its derivative y = f '(x)
Describe The Transformations Required To Obtain The Graph Of The Function F X From The Graph Of The Brainly Com
Graph the function f x ( ) cos(2 ) 1
Graph the function f x ( ) cos(2 ) 1-1) right a unitsWhich set of transformations is needed to graph f(x) = 2sin(x) 3 from the parent sine function?
Integral Of Cos X Add Just A Bit Of Pi
· Get an answer for '`f(x) = cos(x), g(x) = cos(2x)` Describe the relationship between the graphs of f and g Consider amplitude, period, and shifts' and find homework help for other Math questions32 Sketch the graph of g(x) = sin x for xe0°;Relationship between Sine and Cosine graphs The graph of sine has the same shape as the graph of cosine Indeed, the graph of sine can be obtained by translating the graph of cosine by ( 4 n 1) π 2 \frac { (4n1)\pi} {2} 2(4n1)π units along the positive
Graphing Sine & Cosine Functions (II) Author Tim Brzezinski Topic Cosine, Functions, Function Graph, Sine, Trigonometric Functions Interact with the applet below for a few minutes Then answer the questions that follow Questions 1) Consider the function f (x) = sin (x) What are the values of a, b, c, and d for this parent sine function?Trigonometry Graph f (x)=cos (x) f (x) = cos (x) f ( x) = cos ( x) Use the form acos(bx−c) d a cos ( b x c) d to find the variables used to find the amplitude, period, phase shift, and vertical shift a = 1 a = 1 b = 1 b = 1 c = 0 c = 0 d = 0 d = 0 Find the amplitude a aAbout Beyond simple math and grouping (like "(x2)(x4)"), there are some functions you can use as well Look below to see them all They are mostly standard functions written as you might expect
Transcribed image text In the diagram below, the graph of f() = cos x is drawn for xe0%, 3603 3 2 1 O 0 45° 90 135* 180° 225 270° 315 360 1 2 3 31 For which value(s) of x is f decreasing? · $\begingroup$ This is the graph of $\sin x\cos x$ without the greatest integer function $\endgroup$ – Snehil Sinha Jul 13 '17 at 1624 1 $\begingroup$ You must be aware that max value of $\sin x \cos x$ is $\sqrt2$, which after little work means the max value of $\sin x \cos x$ is $\sqrt2$ again $\endgroup$ – Sawarnik Jul 1310 Relative to the graph of y 3sinx, what is the shift of the graph of y 3sinx 3 Ê Ë ÁÁ ÁÁ ÁÁ ˆ ¯ ˜˜ ˜˜ ˜˜?
What Is The Fourier Series Of F X Cos X If 0 X P Quora
Sine Wikipedia
Please be sure to answer the questionProvide details and share your research!We have that f(x)=\sin xx\cos x\implies f(0)=0,\, f(\pi)=\pi and since \sin x > 0 for x\in(0,\pi) f'(x)=x\sin x>0 thus f(x) is strictly increasing on that interval and f(x)>0 We have that f ( x ) = sin x − x cos x f ( 0 ) = 0 , f ( π ) = π and since sin x > 0 for x ∈ ( 0 , π ) f ′ ( x ) = x sin x > 0 thus f ( x ) is strictly increasing on that interval and f ( x ) > 0360°) 33 Write down the period of g
Ppt Graphs Of The Sine And Cosine Functions Powerpoint Presentation Free Download Id 4347
Imath More Exercises Re The Graphs Of The Trigonometric Functions And Their Properties
· I was wrong when I wrote cos x (which is a function pair, so the graph of cos x is no problem) Actually, I wanted to do was make the graph of f (x) = sin x , and I thought I'd just ask a simple question (f(x) = sinx )and, after suggestions, I would complete the restThe trigonometric functions cosine, sine, and tangent satisfy several properties of symmetry that are useful for understanding and evaluating these functions Now that we have the above identities, we can prove several other identities, as shown in the following example Using the properties of symmetry above, we can show that sine and cosine are special types of functionsEstimate the area under the graph of f(x) = 10 cos x from x = 0 to x = {eq}\pi {/eq}/2 using four approximating rectangles and right endpoints
What Are Periodic Functions Expii
Graphing Trig Functions Sin And Cos Andymath Com
Transcribed image text Give an equation of the form f(x) A cos(Bx C) D which could be used to represent the given graph (Note C or D may be zero) 2 TT a TE 311 a) O f(I) = 6 cos 1 1 08(211) b) Of(T) = 6 cos( ) c) O f(t) = 3 cos (217) d) Of(x) = 3 cos(212) e) Of(x) = 3 cos(21 2) 1 1 f) None of the aboveCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyHow can we plot the following three functions f(x) = sin(x) k(x) = cos(x) u(x) = x² for x ∈ 0,1 on a single plot with the help of TikZ?
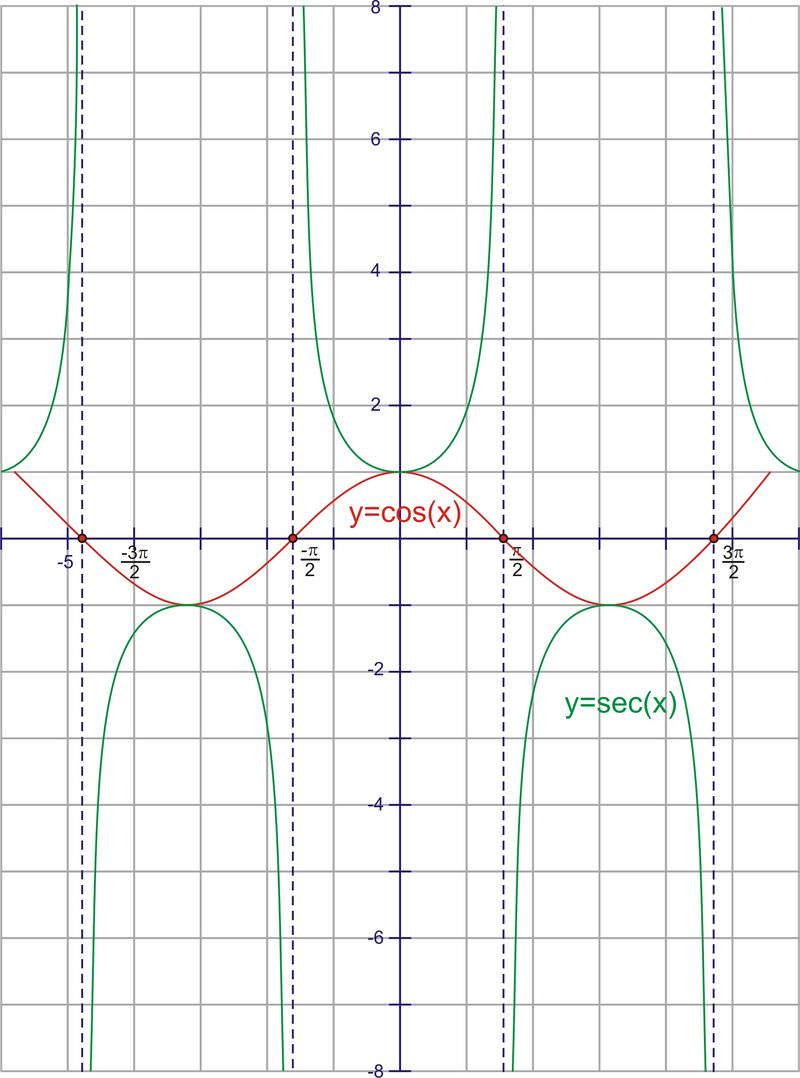
Cosine And Secant Graphs Ck 12 Foundation
Solved A Estimate The Area Under The Graph Of F X 10 Cos X From X 0 To X Jr Z Using Four Approximating Rectangles And Right Endpoints R Course Hero
For real number x, the notations sin x, cos x, etc refer to the value of the trigonometric functions evaluated at an angle of x rad If units of degrees are intended, the degree sign must be explicitly shown (eg, sin x°, cos x°, etc)So if we restrict the domain of f(x) = cosx in this way, we can define cos−1 x 1 −1 0 180° f(x) x f(x) = cos x 1 2 So now we say that our function f(x) = cosx has domain 0 ≤ x ≤ 180 and that it has an inverse, f−1(x) = cos−1 x This inverse function is also written as arccosx So, if the angle xFor a function f (x), the derivative is the limit of h f (x h) − f (x) as h goes to 0, if that limit exists \frac{\mathrm{d}}{\mathrm{d}x}(\cos(x))=\left(\lim_{h\to 0}\frac{\cos(xh)\cos(x)}{h}\right)
Answered Let F X 2 Cos X Complete Parts A Bartleby
Integral Of Cos X Add Just A Bit Of Pi
But avoid Asking for help, clarification, or responding to other answersGet the free "Surface plot of f(x, y)" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Engineering widgets in WolframAlpha · Thanks for contributing an answer to Mathematics Stack Exchange!
Simplify Cos X 2pi
Pin On Different Types Of Functions And Their Graphs
Stack Exchange Network Stack Exchange network consists of 177 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and buildExample The diagram shows a graph of y = cos x for 0° ≤ x ≤ 360°, determine the values of p, q and r Solution We know that cos 180˚ = –1 So, p = –1 We know that for a cosine graph, cos θ = 0 for θ = 90˚ and 270˚ So, θ = 90˚ We know that for a cosine graph, cos θ = 1 for θ = 0˚ and 360˚ So, r = 360˚ ExampleGraph of z = f(x,y) – GeoGebra Graph of z = f(x,y) Graph of z = f(x,y) GeoGebra Create Class GeoGebra Home News Feed Resources
Why Isn T Arccos Cos X Equal To X Mathematics Stack Exchange
Which Is The Graph Of F X Blog Lif Co Id
With your calculator set in radian mode, graph \ (f (x)=\cos 2x\) and \ (y=\cos x\) in the same window, as shown below Both graphs have the same amplitude (\ (1\)) and midline (\ (y=0\)), but the graph of \ (f\) completes two cycles from \ (0\) to \ (2\pi\) instead of oneCosine Function f(x) = cos (x) Graph;The graph of f (x) = x cos x is just the graph of x with 2 πperiodic oscillations on it For reference, let us look at all three graphs
Sin X And Cos X Geogebra
Graph Of Y Sin X Video Trigonometry Khan Academy
1) 3 right 2) 3 left 3) 3 up 4) 3 down 11 Given the parent function p(x) cosx, which phrase best describes the transformation used to obtain the graph of g(x) cos(x a) b, if a and b are positive constants? · f(x) = cos(x) on the interval −2π, 2π (a) Find the xintercepts of the graph of y = f(x) (Enter your answers as a commaseparated list) (b) Find the yintercepts of the graph of y = f(x) (Enter your answers as a commaseparated list) (c) Find the intervals on which the graph of y = f(x) is increasing and the intervals on which the graph ofClick here👆to get an answer to your question ️ The graph of the function cos xcos (x 2) cos^2(x 1) is a
Draw The Graph Of Y Cos X X In 0 2pi Where Repres
2sinxcosx Graph Gamers Smart
Derivative of f (x) = arccos (cos (x)) and its Graph f (x) is a composite function and the derivative is computed using the chain rule as follows Let u = cos (x) Hence f (x) = arccos (u (x)) Apply the chain rule of differentiationCalculus Integral with adjustable bounds example Calculus Fundamental Theorem of Calculus · Sine, Cosine and tangent are the three important trigonometry ratios, based on which functions are defined Below are the graphs of the three trigonometry functions sin x, cos x, and tan x In these trigonometry graphs, xaxis values of the angles are in radians, and on the yaxis, its f(x) is taken, the value of the function at each given angle
Which Function Is Shown On The Graph A F X 1 2 Cos X B F X 1 2 Cos X C F X Brainly Com
The Following Represents The Graph Of The Function Chegg Com
Domain all real numbers Range 1 , 1 Period = 2pi x intercepts x = pi/2 k pi , where k is an integer y intercepts y = 1 maximum points (2 k pi , 1) , where k is an integer minimum points (pi 2 k pi , 1) , where k is an integer symmetry since cos(x) = cos (x) then cos (x) is an even function and its graph is symmetric with respect to theGraph f(x)=2cos(x) Rewrite the expression as Use the form to find the variables used to find the amplitude, period, phase shift, and vertical shift Find the amplitude Amplitude Find the period using the formula Tap for more steps The period of the function can be calculated usingGraph of the function f(x) = x3 − 9x In mathematics, the graph of a function f is the set of ordered pairs (x, y), where f(x) = y In the common case where x and f(x) are real numbers, these pairs are Cartesian coordinates of points in twodimensional space and thus form a subset of this plane In the case of functions of two variables, that
Reflections Of Trig Graphs Examsolutions
Graphs Of The Sine And Cosine Function Precalculus Ii
In order for sin (theta)=cos (theta) both the x and y values must be equal, rather than have the same absolute value Same goes for the next question, while there are other points that are equidistant, you are looking for angles where x=y because x=cos (theta) and y=sin (theta)(a) By graphing the function f (x) = (cos 2 x − cos x) / x 2 and zooming in toward the point where the graph crosses the y axis, estimate the value of lim x → 0 f (x) (b) Check your answer in part (a) by evaluating f (x) for values of x that approach 0 · Sketch a graph of \(f(x)=−2\sin\left(\dfrac{\pi x}{2}\right)\) Solution Let's begin by comparing the equation to the form \(y=A\sin(Bx)\) Step 1 We can see from the equation that \(A=−2\), so the amplitude is 2 \(A=2 \) Step 2 The equation shows
Answered A By Graphing The Function F X Bartleby
Visual Representation Of Factoring A Number Using Trigonometric Functions
Reflection across the xaxis, vertical stretching by a factor of 2, vertical translation 3 units up THIS SET IS OFTEN IN FOLDERS WITHFree functions calculator explore function domain, range, intercepts, extreme points and asymptotes stepbystepGraphs are conventionally plotted with f(x) on the vertical axis The original definition of sin(x) gives it as the length of a vertical segment, so it projects directly onto the graph But cos(x) is defined as the length of a horizontal segment, and to generate the cos graph I had to rotate the circle so that the xaxis was vertical
Solved Select The Graph Of The Function F X 3 Cos X 2 Chegg Com
Rd Sharma Solutions For Class 11 Chapter 6 Graphs Of Trigonometric Functions Download Free Pdf
Sin(x) cos(x) tan(x) asin(x) acos(x) atan(x) sin( deg2rad( x ) ) sqrt(x) PI e e(x) exp(x) ln(x) log(x) abs(x) Infos Bei trigonometrischen Funktionen wird das Bogenmaß verwendet Sinus um Gradmaß Konstante von Pi (ca 3,) Konstante der Eulerschen Zahl (ca 2,718) Die EFunktion e^x Betragsfunktion abs(1) = 1; · Explanation To graph f (x) = 1 cos(x), start working on it's Parent Function f (x) = cos(x) first Make a table of values for f (x) = cos(x) and f (x) = 1 cos(x) For x, consider the values 0, π 2,π, 3π 2 and 2π If you examine Col 4 and Col 5, you see that the difference is 1Graphs of trigonometric functions The graph of the cosine function f (x) = cos x To draw the graph of the cosine function divide the unit circle and x axis of a Cartesian coordinate system the same way as when drawing the sine function
If F X Cosx And G X Sin X Then Graph Of F Xd
How Do You Graph Y Cosx 3 Socratic
· Let R be the region in the first quadrant enclosed by the graph of f(x) = sqrt cosx, the graph of g(x) = e^x, and the vertical line pi/2, as shown in the figure above (a) Write but do not evaluate, an integral expression that gives the area of R (b) Find the volume of the solid generated when R is revolved about the xaxis (c) Region R is the base of a solid whose crosssections
Graphing Sine And Cosine Trigonometry
A 72 Identifying The Equation Of A Trigonometric Graph 1 Write
Graph Of Arccos Cosx Graph Of Cos Inverse Cosx Arccos Cos X Cos 1 Cos X X Youtube
How To Graph Sine And Cosine Functions 15 Steps With Pictures
Bestmaths
Which Function Is Graphed Below Wmx F X Cos X F X Cos X X Sin Brainly Com
Draw The Graph Of Y Cos X X In 0 2pi Where Repres
Find Critical Points Of F X Max Sinx Cosx X In 0 2pi Youtube
Ppt Trig Graphs Powerpoint Presentation Free Download Id
Derivatives Of Sin X And Cos X Video Khan Academy
Answered Let F X 2 Cos X Complete Parts A Bartleby
What Is The Amplitude Of Y Cos 3x And How Does The Graph Relate To Y Cosx Socratic
Graphs Of The Sine And Cosine Function Precalculus Ii
The Range Of Function F X Cos X
Graphs Of Trigonometric Functions Sine Cosine Tangent Etc
Describe The Transformations Required To Obtain The Graph Of The Function F X From The Graph Of The Brainly Com
Math 127 Final Review Sheet Graphing Angles And Radian Measure
How Do You Graph Y Cosx 3 Socratic
Graphing Y Sinx And Y Cosx In Degrees Youtube
Graph Of F X Sin X Cos X Youtube
Graph Transformations Ppt Download
Im Currently Learning Taylor Polynomials In The Picture The Red Line Is The Graph Of F X Cos X The Blue Line Is The 5th Degree Taylor Polynomial Centered At X 0 For This
Graphing Trig Functions Sin And Cos Andymath Com
Solved A Estimate The Area Under The Graph Of F X 10 Cos X From X 0 To X Jr Z Using Four Approximating Rectangles And Right Endpoints R Course Hero
Graph The Function For Two Periods F X 5 Cos X Chegg Com
Unit 7 Trigonometric Functions Ppt Download
F X C O S X G R A P H Zonealarm Results
Solved A Estimate The Area Under The Graph Of F X 10 Cos X From X 0 To X Jr Z Using Four Approximating Rectangles And Right Endpoints R Course Hero
F X Cos X Omicron Math And Engineering
In The Diagram Below The Graph Of Fx Cos X Is Dra Gauthmath
Generating The Graph Of F X Cosx Geogebra
Graphs Of Trigonometric Functions Sine Cosine Tangent Etc
The Derivative Of F X Sin X Is G X Cos X Geogebra
Examples With Trigonometric Functions Even Odd Or Neither Video Lessons Examples And Solutions
Find The Math 8 Math Th Degree Taylor Polynomial Centered At Math A 0 Math For The Function Math F X Cos X Math Graph Math F Math Together With The Taylor Polynomials Math T 2 T 4 T 6 T 8 Math In
Pp 6 If F I 1 1 F X Cos X Fin 0 8 0 6 0 4 2 Chegg Com
What Is The Range Of Cos X
Proof Of The Derivative Of Cos X Video Khan Academy
Cosine And Secant Graphs Ck 12 Foundation
How Do You Graph The Derivative Of F X Cos X Socratic
Intersection Points Of Y Sin X And Y Cos X Video Khan Academy
Transformation Of Cos X To Sin X Via Cos X Frac Pi 2 Sin X Mathematics Stack Exchange
Solved Nsider The Following F X 7 Cos X 5 G X Chegg Com
Example 13 Find Area Bounded By Y Cos X X 0 2pi Examples
The Number Of Points Where F X Sin X Cosx Where Denotes The Greatest Integer Fun Youtube
What Are Periodic Functions Expii
Is This The Correct Graph Of F X Cos X 45 Askmath
Answered On The Graph Of F X Cos X And The Bartleby
Draw The Graph Of Y Sin X Cos X X In 0 2pi
Notes On Topics Of Algebra Notes
Misc 19 Area Bounded By Y Axis Y Cos X Y Sin X Miscellaneous
Find The Values Of X For Which F X Sqrt Sin X Cos X Is Def
0 件のコメント:
コメントを投稿